Submitted by admin on

General Overview

According to Kitajima et al. and Watanabe et al. influenza A viruses are members of the family Orthomyxoviridae, which is comprised of enveloped viruses with segmented, negative-sense RNA genomes. Based on the antigenicity of the two surface glycoproteins, hemagglutinin (HA) and neuraminidase (NA), influenza A viruses are currently divided into 16 HA and 9 NA subtypes, designated as H1-H16 and N1-N9. Over the past century, viruses of the H1N1, H2N2, H3N2, and H1N2 subtypes have circulated in humans. Additionally, new subtypes such as H5N1 and H7N9 have been recently isolated from human as well as poultry. Influenza A virus is one of the most common causes of human respiratory infections and the most significant because they cause high morbidity and mortality. Transmission of influenza can be achieved via environmental reservoirs or human-to-human communication.

Summary Data

Murphy et al.  intranasally challenged adult human volunteers with influenza A (H1N1) California/10/78 cold-adapted viruses. Infection was defined as virus recovery and/or antibody response.

Murphy et al. challenged adult human volunteers with influenza A (H3N2) Washington/897/80 avian-human reassortant viruses via the intranasal route. Infection was defined as virus isolation and/or antibody response.

Fan et al. exposed six-week-old SPF BALB/c mice (five mice/dose) intranasally with a highly pathogenic avian influenza A (H5N1) virus (DKGX/35 strain).

 

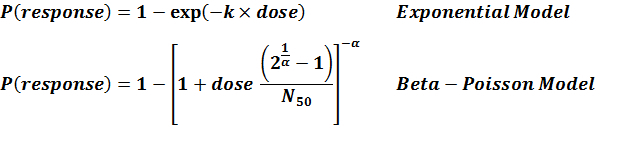
Exponential and betapoisson model.jpg

D # of Doses Agent Strain Dose Units Host type Μodel Optimized parameters Response type Reference
257 4 H1N1,A/California/10/78 attenuated strain TCID50 human beta-Poisson a = 9.04E-01

LD50/ID50 = 1.25E+06
N50 = 1.25E+06		 infection Murphy et al.
257, 258 9 H1N1,A/California/10/78 attenuated strain,H3N2,A/Washington/897/80 attenuated strain TCID50 human beta-Poisson a = 5.81E-01

LD50/ID50 = 9.45E+05
N50 = 9.45E+05		 infection

Murphy et al.
258 5 H3N2,A/Washington/897/80 attenuated strain TCID50 human beta-Poisson a = 4.29E-01

LD50/ID50 = 6.66E+05
N50 = 6.66E+05		 infection Fan et al.
259 6 H5N1, DKGX/35 strain EID50 mice exponential
k = 1.09E-02
LD50/ID50 = 6.38E+01
 		 death DeDiego et al.

Optimization Output for Exp. 257:

Experiment ID: 257

# of Doses: 4

Agent Strain: H1N1,A/California/10/78 attenuated strain

Dose Units: TCID50

Host type: human

Μodel: beta-Poisson

Optimized parameters: 

a = 9.04E-01
LD50/ID50 = 1.25E+06
N50 = 1.25E+06

human/H1N1 A/California/10/78 attenuated strain model data (Murphy et al.)
Dose Infected Non-infected Total
63095.73 0 15 15
630957.3 4 7 11
6309573 19 3 22
63095734 24 1 25

 

Goodness of fit and model selection
Model Deviance Δ Degrees 
of freedom		 χ20.95,1 
p-value 		χ20.95,m-k 
p-value
Exponential 23.6 21.5 3 3.84 
3.47e-06 		7.81 
3.09e-05
Beta Poisson 2.02 2 5.99 
0.365
Beta-Poisson fits better than exponential; cannot reject good fit for beta-Poisson.

 

Optimized parameters for the beta-Poisson model, from 10000 bootstrap iterations
Parameter MLE estimate Percentiles
0.5% 2.5% 5% 95% 97.5% 99.5%
α 9.04E-01 4.20E-01 4.91E-01 5.45E-01 1.73E+03 2.57E+04 2.19E+05
N50 1.25E+06 5.27E+05 6.43E+05 7.19E+05 2.39E+06 2.74E+06 3.45E+06

 

Parameter scatter plot for beta Poisson model ellipses signify the 0.9, 0.95 and 0.99 confidence of the parameters.

beta Poisson model plot, with confidence bounds around optimized model

Optimization Output for Exp. 257, 258:

Best Fit

Experiment ID: 257, 258

# of Doses: 9

Agent Strain: H1N1,A/California/10/78 attenuated strain,H3N2,A/Washington/897/80 attenuated strain

Dose Units: TCID50

Host type: human

Μodel: beta-Poisson

Optimized parameters: 

a = 5.81E-01
LD50/ID50 = 9.45E+05
N50 = 9.45E+05

Pooled dose response data (Murphy et al.)
Dose Infected Non-infected Total
63095.73 0 15 15
1E+05 2 10 12
630957.3 4 7 11
1E+06 8 5 13
6309573 19 3 22
1E+07 16 3 19
31622777 16 4 20
63095734 24 1 25
1E+08 19 0 19

 

Goodness of fit and model selection
Model Deviance Δ Degrees 
of freedom		 χ20.95,1 
p-value 		χ20.95,m-k 
p-value
Exponential 64 55.4 8 3.84 
9.68e-14 		15.5 
7.63e-11
Beta Poisson 8.56 7 14.1 
0.285
Beta-Poisson fits better than exponential; cannot reject good fit for beta-Poisson.

 

Optimized parameters for the beta-Poisson model, from 10000 bootstrap iterations
Parameter MLE estimate Percentiles
0.5% 2.5% 5% 95% 97.5% 99.5%
α 5.81E-01 3.61E-01 3.98E-01 4.24E-01 9.15E-01 1.02E+00 1.36E+00
N50 9.45E+05 4.38E+05 5.28E+05 5.72E+05 1.62E+06 1.79E+06 2.23E+06

 

Parameter scatter plot for beta Poisson model ellipses signify the 0.9, 0.95 and 0.99 confidence of the parameters.

beta Poisson model plot, with confidence bounds around optimized model

Optimization Output for Exp. 258:

Experiment ID: 258

# of Doses: 5

Agent Strain: H3N2,A/Washington/897/80 attenuated strain

Dose Units: TCID50

Host type: human

Μodel: beta-Poisson

Optimized parameters: 

a = 4.29E-01
LD50/ID50 = 6.66E+05
N50 = 6.66E+05

human/H3N2, A/Washington/897/80 attenuated strain model data (Fan et al.)
Dose Infected Non-infected Total
1E+05 2 10 12
1E+06 8 5 13
1E+07 16 3 19
31622777 16 4 20
1E+08 19 0 19

 

Goodness of fit and model selection
Model Deviance Δ Degrees 
of freedom		 χ20.95,1 
p-value 		χ20.95,m-k 
p-value
Exponential 39.1 34.8 4 3.84 
3.66e-09 		9.49 
6.79e-08
Beta Poisson 4.26 3 7.81 
0.235
Beta-Poisson fits better than exponential; cannot reject good fit for beta-Poisson.

 

Optimized parameters for the beta-Poisson model, from 10000 bootstrap iterations
Parameter MLE estimate Percentiles
0.5% 2.5% 5% 95% 97.5% 99.5%
α 4.29E-01 2.14E-01 2.58E-01 2.83E-01 7.58E-01 8.71E-01 1.20E+00
N50 6.66E+05 1.36E+05 2.19E+05 2.68E+05 1.63E+06 1.87E+06 2.49E+06

 

Parameter scatter plot for beta Poisson model ellipses signify the 0.9, 0.95 and 0.99 confidence of the parameters.

beta Poisson model plot, with confidence bounds around optimized model

Optimization Output for Exp. 259:

Experiment ID: 259

# of Doses: 6

Agent Strain: H5N1, DKGX/35 strain

Dose Units: EID50

Host type: mice

Μodel: exponential

Optimized parameters:
k = 1.09E-02
LD50/ID50 = 6.38E+01
 

mice/ H5N1,DKGX/35 strain model data (DeDiego et al.)
Dose Dead Survived Total
10 1 4 5
100 3 2 5
1000 5 0 5
1E+04 5 0 5
1E+05 5 0 5
1E+06 5 0 5

 

Goodness of fit and model selection
Model Deviance Δ Degrees 
of freedom		 χ20.95,1 
p-value 		χ20.95,m-k 
p-value
Exponential 0.498 0.0917 5 3.84 
0.762 		11.1 
0.992
Beta Poisson 0.406 4 9.49 
0.982
Exponential is preferred to beta-Poisson; cannot reject good fit for exponential.

 

Optimized k parameter for the exponential model, from 10000 bootstrap iterations
Parameter MLE estimate Percentiles
0.5% 2.5% 5% 95% 97.5% 99.5%
k 1.09E-02 3.14E-03 3.29E-03 4.56E-03 2.78E-02 3.48E-02 5.42E-02
ID50/LD50/ETC* 6.38E+01 1.28E+01 1.99E+01 2.49E+01 1.52E+02 2.11E+02 2.21E+02
*Not a parameter of the exponential model; however, it facilitates comparison with other models.

 

Parameter histogram for exponential model (uncertainty of the parameter)

Exponential model plot, with confidence bounds around optimized model

Recommended Model

It is recommended that the pooled experiments 257 and 258 should be used as the best dose-response model. Both strains are common in human outbreaks. The pooling narrows the range of the confidence region of the parameter estimates and enhances the statistical precision.

Advanced Dose Response Model

Incorporating the time postinoculation into the classical dose-response models for microbial infection generates a class of time-dose-response (TDR) models. The parameter k in the exponential dose-response model (equation 1) and the parameter N50 in the beta-Poisson model (equation 2) were set equal to functions of time that represent in vivo bacterial kinetics. Equations 1-2 with candidate G(t; θ,…) were fit to the time-dependent dose response data from experiment 259. The beta-Poisson TDR model (equation 2) incorporating an exponential-inverse-power distribution provided the best fit to the data. In the following figure, the best TDR models are plotted to compare with the observed mortalities, according to Kitajima et al. (under review). As shown, the clear difference between the different times postinoculation gives a visible representation of the quantified results that the modification added to the classical models has a substantial effect on the dose response.

The best-fit TDR model (curves) compared to observed mortalities against doses (symbols) from experiment 259.

Equation influenza.png

Summary

The pooling results indicate that the human responses to HIN1 and H3N2 viruses may have similar patterns.

References

  • Kitajima, M. ., Huang, Y. ., Watanabe, T. ., Katayama, H. ., & Haas, C. N. (2011). Dose–response time modelling for highly pathogenic avian influenza A (H5N1) virus infection. Letters in Applied Microbiology, 53, 438–444. Retrieved from https://onlinelibrary.wiley.com/doi/full/10.1111/j.1472-765X.2011.03128.x
  • Watanabe, T. ., Bartrand, T. A., Omura, T. ., & Haas, C. N. (2012). Dose-Response Assessment for Influenza A Virus Based on Data Sets of Infection with its Live Attenuated Reassortants. Risk Analysis, 32, 555–565. Retrieved from https://onlinelibrary.wiley.com/doi/full/10.1111/j.1539-6924.2011.01680.x
  • Murphy, B. R., Clements, M. L., Madore, H. P., Steinberg, J. ., O’Donnell, S. ., Betts, R. ., … Maassab, H. F. (1984). Dose Response of Cold-Adapted, Reassortant Influenza A/California/10/78 Virus (H1N1) in Adult Volunteers. Journal of Infectious Diseases, 149, 5. Retrieved from https://www.ncbi.nlm.nih.gov/pubmed/6726007
  • Murphy, B. R., Clements, M. L., Tierney, E. L., Black, R. E., Stienberg, J. ., & Chanock, R. M. (1985). Dose Response of Influenza A/Washington/897/80 (H3N2) Avian-Human Reassortant Virus in Adult Volunteers. The Journal of Infectious Diseases, 152, 1. Retrieved from http://www.jstor.org/stable/30104663?seq=1#page_scan_tab_contents
  • Fan, S. ., Deng, G. ., Song, J. ., Tian, G. ., Suo, Y. ., Jiang, Y. ., … Chen, H. . (2009). Two amino acid residues in the matrix protein M1 contribute to the virulence difference of H5N1 avian influenza viruses in mice. Virology., 384, 1. Retrieved from https://www.sciencedirect.com/science/article/pii/S0042682208007964
  • DeDiego, M. L., Pewe, L. ., Alvarez, E. ., Rejas, M. T., Perlman, S. ., & Enjuanes, L. . (2008). Pathogenicity of severe acute respiratory coronavirus deletion mutants in hACE-2 transgenic mice. Virology, 376, 379–389.